One of the biggest findings in our largest-ever politics survey of Americans was that the public is more polarized than at any time in recent history. To illustrate, we included an interactive data visualization of the blue and red “mountains” of Americans, representing Democrats and Republicans and how their views have changed over time. This unique interactive does not include a labeled y (vertical) axis, as many are used to seeing in charts. This was an intentional decision to avoid miscommunication about the meaning of the area represented by the “mountains.”
In this case, the “mountains” represent the distribution of the American public, from consistently conservative to consistently liberal, according to how they answered a series of 10 questions about their political views. To create the distribution, we used their responses to assign them an ideological consistency score – this is the x (horizontal) axis. The interactive is actually a “smoothed” histogram of these scores, where points along the curve correspond to averages of adjacent scores. (Find a more detailed explanation here.)
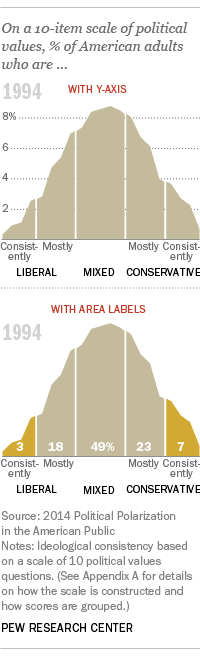
In a histogram, it’s the area under the curve that matters, not the height of any specific point. The total area under the curve is equal to 100% of respondents, but we are most interested in where respondents fall along the horizontal axis. In other static versions of this chart in the report, we display this information in more annotated ways.
The chart on the right shows what the distribution of Americans overall would look like with a y-axis. Note that the percentages on the y-axis do not tell us much about the area under the curve, and could actually lead to confusion about the true volume of the “peak” and “tails.” Below, we’ve labeled the segments of the area under the curve, which shows a much clearer picture of the distribution of all Americans on this scale. While we could have included this information in the animation, these added layers would overwhelm the visual impact of the changing distribution.
The primary data story of the moving “mountains” animation is the changing shape (spread and central tendency) of the distribution. And as with any visualization, we had to make some tradeoffs in terms of how much information we could show. However, we do believe it’s important to provide the data behind our graphics, and in this case, a number of static charts in the report provide greater detail about the area of the curve using different metrics and more appropriate visualizations for these data.